Or, the Many Uses of Uselessness
One of the joys of being married to a pure mathematician—other than finding coffee-stained notebooks full of integrals lying around the flat—is hearing her try to explain her job to other people.
“Are there…uh… a lot of computers involved?”
“Do you write equations? I mean, you know, long ones?”
“Do you work with really big numbers?”
No, sometimes, and no. She rarely uses a computer, traffics more with inequalities than equations, and—like most researchers in her subfield—considers any number larger than 5 to be monstrously big.
Still, she doesn’t begrudge the questions. Pure math research is a weird job, and hard to explain. (The irreplaceable Jordy Greenblatt wrote a great piece poking fun at the many misconceptions.)
So, here’s this teacher’s feeble attempt to explain the profession, on behalf of all the pure mathematicians out there.
Q: So, what is pure math?
A: Picture mathematics as a big yin-yang symbol. But instead of light vs. dark, or fire vs. water, it’s “pure” vs. “applied.”
Applied mathematicians focus on the real-world uses of mathematics. Engineering, economics, physics, finance, biology, astronomy—all these fields need quantitative techniques to answer questions and solve problems.
Pure mathematics, by contrast, is mathematics for its own sake.
Q: So if “applied” means “useful,” doesn’t it follow that “pure” must mean…
A: Useless?
Q: You said it, not me.
A: Well, I prefer the phrase “for its own sake,” but “useless” isn’t far off.
Pure mathematics is not about applications. It’s not about the “real world.” It’s not about creating faster web browsers, or stronger bridges, or investment banks that are less likely to shatter the world economy.
Pure math is about patterns, puzzles, and abstraction.
It’s about ideas.
It’s about the other ideas that come before, behind, next to, or on top of those initial ones.
It’s about asking, “Well, if that’s true, then what else is true?”
It’s about digging deeper.
Q: You’re telling me there are people out there, right this instant, doing mathematics that may never, ever be useful to anyone?
A: *glances over at wife working, verifies that she’s not currently watching Grey’s Anatomy*
Yup.
Q: Um… why?
A: Because it’s beautiful! They’re charting the frontiers of human knowledge. They’re no different than philosophers, artists, and researchers in other pure sciences.
Q: Sure, that’s why they’re doing pure math. But why are we paying them?
A: Ah! That’s a trickier question. Let me distract you from it with a rambling story.
In the 19th century, mathematicians became obsessed with proof. For centuries, they’d worked with ideas (like the underpinnings of calculus) that they knew were true, but they couldn’t fully explain why.
So at the dawn of the 20th century a few academics, living on the borderlands between math and philosophy, began an ambitious project: to prove everything. They wanted to put all mathematical knowledge on a firm foundation, to create a system that could—with perfect accuracy, and utter permanence—separate truth from falsehood.
This was an old idea (Euclid put all of planar geometry on a similar footing 2000 years earlier), but the scope of the project was new and monumental. Some of the world’s intellectual titans spent decades trying to explore the rigorous, hidden meanings behind statements like “1 + 1 = 2.”
Can you imagine anything more abstract? Anything more “pure”? Curiosity was their compass. Applications could not have been further from their minds.
Q: So? What happened?
A: The project failed.
Eventually, the philosopher Kurt Gödel proved that no matter what axioms you choose to start with, any system will eventually run into statements that can’t be proven either way. You can’t prove them true. You can’t prove them false. They just… are.
We call these statements “undecidable.” The fact is, many things can be proven, but some things never can.
Q: Ugh! So it was just a massive waste of time! Pure maths is the worst.
A: Oh, I suppose you’re right.
Of course, the researchers tried to salvage something from the wreckage. Building on all this work, one British mathematician envisioned a machine that could help us decide which mathematical statements are true, false, or undecidable. It would be an automatic truth-determiner.
Q: Did they ever build it?
A: Yeah. The guy’s name was Alan Turing. Today we call those machines “computers.”
Q: *stares blankly, jaw slowly unhinging*
A: Exactly.
This enormous project to prove everything—one of the purest mathematical enterprises ever undertaken—didn’t just end with a feeble flicker and a puff of smoke. Far from it.
Sure, it didn’t accomplish its stated goals. But by clarifying (and, at times, revolutionizing) ideas like “proof,” “truth,” and “information,” it did something even better.
It gave us the computer, which in turn gave us… well… the world we know.
Q: So the pure mathematics being done today might, someday, give us a new application as transformative as the computer?
Maybe.
But you shouldn’t hold any specific piece of work to that standard. It won’t meet it. Paper by paper, much of the pure math written this century will never see daylight. It’ll never get “applied” in any meaningful sense. It’ll be read by a few experts in the relevant subfield, then fade into the background.
That’s life.
But take any random paper written by an early 20th-century logician, and you could call it similarly pointless. If you eliminated that paper from the timeline, the Jenga tower of our intellectual history would remain perfectly upright. That doesn’t make those papers worthless, because research isn’t a collection of separable monologues.
It’s a dialogue.
Every piece of research builds on what came before, and nudges its readers to imagine what might come next. Those nudges could prove hugely valuable. Or a little valuable. Or not valuable at all. It’s impossible to say in advance.
In this decades-long conversation, no particular phrase or sentence is necessarily urgent. Much will be forgotten, or drift into obscurity. And that’s all right. What’s vital is that the conversation keeps on flowing. People need to continue sharing ideas that excite them, even—or perhaps especially—if they can’t quite explain why.
Q: So, pure maths… come for the pretty patterns, stay for the revolutionary insights?
A: That about covers it.






Could the same question apply to theoretical physics? Why are we paying theoretical physicists to do blue sky research?
https://www.youtube.com/watch?v=O577tYW0AQI&lc=01nAY9MdTMV8DRyFNpkoWufmGjWC9PaZXk7180yUTMs
I think it would suffice to answer why we pay people to do theoretical research or something like that.
* youtube.com/watch?v=O577tYW0AQI&lc=01nAY9MdTMV8DRyFNpkoWufmGjWC9PaZXk7180yUTMs
Yeah, I think this argument applies quite directly to all basic science. (“Basic” is to science what “pure” is to math.) The idea is that learning more about our universe and its mysteries generally pays huge rewards in the long term. (This is essentially the mission statement for the NSF.)
As to justifying humanities research… I think you need a different set of arguments, perhaps more along the lines of what John Cowan suggests below.
Nice!
There’s another line of defense, however. Pure math, like the arts, is not a means to an end: it is itself part of the end toward which all the applications are means.
Okay, so here’s another question. Why do we pay pure mathematicians a lot? Many of the pure math folks I know are 100% unemployable in any other field. They would be happy to sit around and ponder the mysteries of the prime numbers for half of what they currently make, right?
Yeah, I like that argument too, John. It’s nice to think that our intellectual activity is the ends, and our economic activity is merely a means (rather than the other way around). Of course, that boils down to a debate about values, which is perhaps most easily sidestepped by my argument above (that theoretical research has HUGE economic benefits, they’re just very unpredictable and sometimes far-off).
As to your question, sanchomundo… I dunno. I know lots of pure math PhD’s who left the field, spent 6 months retraining as programmers, and now make about 4-5x the typical postdoc salary (or 2-3x the typical professor salary). I’m often stumped as to why so many PhD’s scratch out a meager living in the hyper-competitive academic job market, rather than taking their skills and education to where they’ll be more amply rewarded.
Why, because they are taught by tenured faculty who had less trouble finding jobs, and who constantly din into their ears that leaving academia equals failure.
Reblogged this on An Accountant and a Mum and commented:
I want to remember this especially if either of my daughters ever become mathematicians!
Reblogged this on A Philosopher Muses.
Reblogged this on P R S and commented:
Mathematics…
Very well said.
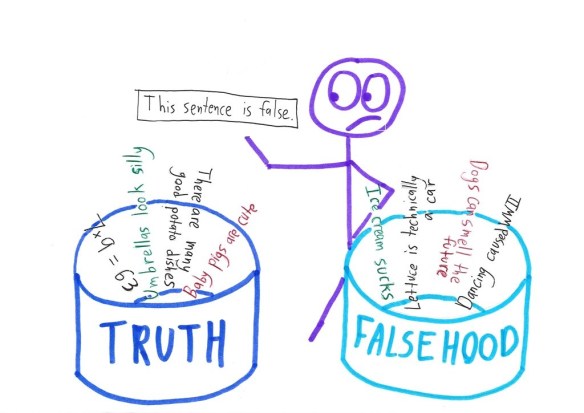
A great explanation, thank you. I especially love the line “this sentence is false”!
Yeah, that actually IS the fundamental paradox that Godel exploited!
It turns out that you can make paradoxical statements like this in every language, including mathematical languages. And this is the crowbar you use to break apart any system of proof.
(Another famous version of this paradox is “the set of all sets that don’t contain themselves.” Such as set can neither contain itself, nor NOT contain itself!)
His sentence wasn’t quite “this sentence is false”, which has no well-defined truth value.
It was more like “the truth of this sentence cannot be PROVED in this system”. If the sentence is provable, then it’s also false, and the system breaks from a self-contradiction. So it must be unprovable, and therefore, it’s also true!
So any axiom system sufficiently powerful to admit such a sentence is either inconsistent, or incomplete when it comes to proving true statements. And Godel proved that natural-number arithmetic was sufficiently powerful to say that, so any axiom system that can do natural-number arithmetic runs into this problem.
“So it must be unprovable”
…if the system is consistent, that is.
> no matter what axioms you choose to start with, any system will eventually run into statements that can’t be proven either way.
This is not true. There are well-known logical theories that are decidable. Take the Presburger arithmetic, for example. Godel’s theorem is about logical theories that have sufficient expressive power.
Yes, but the standard is fairly low. You just have to admit the integers, nothing more complex than that.
It’s still a false claim. Moreover, the Presburger arithmetic has the integers. It does not have multiplication.
Thanks for the correction.
In explaining concepts at an elementary level, I find there’s a trade-off between accuracy and clarity. I’m pitching this at a very non-technical level, and therefore focusing on clarity.
I don’t see a simple edit that would improve the accuracy without harming the clarity, but let me know if you see one.
Why not change “no matter what axioms you choose to start with,” say “any system that can model basic arithmetic”
I think that’s too technical. I’m not necessarily assuming that the reader knows, going in, what an axiomatic system is, much less what the verb “model” would mean in the phrase “model basic arithmetic.”
But, if you take in account that integers, come from naturals, and naturals come from cardinals, and cardinals come from set theory, you can then think of the Gödel´s theorem…
Ben,
I commonly give talks to general audiences. When talking about Godel, I simply state that Godel showed it is possible to have mathematical statements that are either true or false, but it is impossble to prove which of the two options is the case. Turing then proceeded to construct actual examples. It saves an awful lot of nitpicking.
Ah, the good old Math that No One Can Use vs Tainted Math debate.
Interesting post, but I have a question: Isn’t the ultimate point of pure math for it to perhaps at some point become applied math?
Nah, what would be the fun of that? =D As a pure mathematician I have never asked myself ‘will this be ever useful’. I care more about finding the intracacies and patterns found in the world around us then to worry about whether it has actual applications. Sure, one day it might become helpful, but that’s not the goal.
Sounds right to me!
I’d expect that most pure mathematicians feel the same way. As for the surrounding world, I think we ought to leave y’all to explore, not just ’cause it makes you happy, but because who knows where the work will lead a hundred years from now. It’s long-term R&D for us, fun for you.
We need to realize that first computers were built WHILE Truing was alive and INDEPENDENTLY from his research. He helped design some computers, but his main contributions are theoretical (yes, “Turing machines” are not even nearly what might seem from that “Imitation Game” superhero movie). Saying that he invented computers is ignorant and disrespectful. His inventions are far more fundamental.
The author never said that Turing invented computers. He said that his theoretical contributes were one of the key necessities for computers to be where they are today. There were a great many minds before computers came to realisation, but no one would argue that one of those great minds was Turing. Without his contribution (or without the contribution of any additional person) computers as we know today wouldn’t exist. (The concept might exist, but we’d be quite a few years further behind)
Turing’s work was on computability, ie that which can be achieved by the manipulation of symbols and numbers. He did this work in the 1930’s in the wake of Godel’s work. Electronic computers were not in his thinking. Turing’s work had little to no effect on computers as we know then today.
The software influences the hardware and vice versa. Think again if you say it had little effect.
@Andrey Stavitskiy. I can think of no overwhelmingly significant ways in which Turing’s work has advanced computers. This is not to say his work is not without note, though many of the results we know to day came from others extending his work. For instance, the Busy Beaver numbers or the un-computability of the Halting probability, although remarkable results, have not affected computers or computing significantly. Some of his ideas are used in NP-Completeness, but again how much has this really affected computing? His ideas may affect computing in the future but have not to date, I do not buy your argument that he affected software significantly.Turing was working in the wake of Godel and others and only worked in the area for a short time; electronic computing was not in his thinking.
Turing work was on computable numbers and I think the Turing machine concept is remarkable. To say he has not affected my thinking would be untrue, but he is not an Einstein or a Newton.
Saying that “Turing’s work had little to no effect on computers as we know then today” is simply wrong. Turing may not have invented a practical computer himself, but with Turing Machines, he gave us a theoretical model that defines what a computer / computability is, and what the limits of a computer are (at least of a computer as we know it). Take music history as an analogy. Look up Franco of Cologne (considered the founder of modern musical notation). He didn’t “invent modern music”. He’s not nearly as famous as Mozart or Beethoven. But he has a profound effect on how we work with and understand music to this day. Similarly, Turing is not Von Neumann or Zuse, but his effect on how we understand computers will probably last longer than will theirs.
I tried to walk a line in my phrasing between simplifying and oversimplifying. But I appreciate that you feel like I crossed it.
I’ll take a look and see if I can find a good edit that will capture the main thrust of my argument (that Turing’s contributions were enormous, and were impossible without earlier work by Russell, Godel, and the like) without making a reductive claim, such as that Turing was the sole inventor of the modern computer.
Lovely post! I shall be quoting some of the responses when I’m asked similar questions. Thanks for making life that little bit easier!
Hello,
I’m a member of an Italian group dedicated to scientific communication – you can find us at http://www.italiaxlascienza.it/main although the website is completely in italian. I really liked this post (the whole blog, to be honest, but this post in particular) and would like to translate it in italian for our readers (crediting you as the author, of course). Please tell me if I can start translating.
Thanks for reading, and go right ahead! Send me the link when you’re done!
Here you are! Thank you 🙂
http://italiaxlascienza.it/main/2015/03/perche-paghiamo-i-matematici-puri/
Pure Brilliance. Excellent. Has had the effect of turning a technologist turned law enforcer to reflect purely on the abstracts and ponder over how purity and excellence tend to prove the same point!
This question is being looked at in more than just a superficial form. The discussion is difficult and come downs to what we consider to be of of cultural value. Mathematicians should be aware of this because some of these ideas are affecting the way governments are funding Mathematics research, and thus Mathematics in general.
One of the seminal works is :- “The Social Life of Mathematics” by Sal Restivo http://logica.ugent.be/philosophica/fulltexts/42-2.pdf
The crux of the argument is – that Mathematics does not hold the secrets of the universe that will unfold the more do of it, but that it is a social construction that was invented for practical needs. It has produced many things of fairly universal cultural worth and with any human activity specialization has occurred. With repeated specialization can come isolation from the original culture and the formation of value systems that have have no relevance to anyone else. In essence it claims some of the more obscure areas of Pure Mathematics are isolated subcultures that have no worth other than to themselves. The same phenomena can be seen in the arts with what some would claim are absurd pieces of art, music or dance.
It further claims a sub-discipline of pure mathematics must have a connection, either directly or indirectly, to the outside world of maths problems, else it will eventually have no cultural value other than to those within the sub-discipline.
I don’t understand if you back up this argument or not. Point is: you quote “a sub-discipline of pure mathematics must have a connection, either directly or indirectly, to the outside world of maths problems, else it will eventually have no cultural value other than to those within the sub-discipline.”. Mathematical theories have many part that do not apply directly. Think of every differential equation you can write. Every one of them is valuable in math, but I doubt that EVERY one of them is valuable outside.
The most difficult thing to accept is that even dead ends and failures are extremely valuable in mathematics and in science in general. How can one know if a sub-discipline will or will not have a connection with the world outside of mathematics without trying?
In the end math answer questions. It is common that pure math helps other pure math and expand the view on the problems. It ought to make an impact on other disciplines as well.
Differential equations still have a connection, even if indirect, to the world of Maths problems. The argument is that specializations can become so insular that they have no longer any connection to the world of maths problems, or anyone else for that matter. They become isolated sub-cultures that develop their own value systems and cultural norms. They become no different that chess culture, golf culture of tennis culture; enjoyable pursuits but having little intrinsic value otherwise. Anything they produce will only of cultural value to their sub-culture. The chances of the producing anything of value to .the outside culture is remote; Maths does not hold the secrets of the universe. It could be argued they are in fact no longer doing Mathematics, but doing puzzles.
To avoid this, areas periodically need to have a connection, regardless of how indirect, to the world of Math problems.
These ideas are gaining a hold in high places and Mathematicians better be aware of this.
What is this “world of Math problems” that you speak of? What do you mean by these words?
I’m certainly willing to believe that academic cultures can turn from stars (projecting light and heat outwards) into black holes (collapsing under their own gravity, and simply sucking resources, providing nothing of value back to the world).
But is there a reliable way to tell the difference?When has a field lost its connection with reality? How long is too long without furnishing a useful application?
These questions are difficult to answer, and I think society is better off giving a lot of leeway to researchers, as long as the community agrees that a topic is intellectually interesting.
Godel isn’t the only example of pure maths finding an application after a long delay. Hyperbolic geometry seemed like a useless “puzzle” until it helped describe the curvature of the universe. GH Hardy famously described number theory as unlikely to find any warlike application; it’s now the basis of all computer encryption.
Gwyn, your argument might apply more directly to certain fields of research in the humanities. I don’t know nearly enough about humanities departments to make the case either way.
It seems to me that you have no idea to how math is built. When you mentioned “the outside world of math problems” I thought you meant problems outside of mathematics but I was wrong. You meant exactly the problems that mathematics is made of. Differential equations are math problems. Pretty valuable at that. They are not some far fetched problems that have some connections with mathematics. They are pretty basic and pretty old. When I hear “math problems” I think of things that most people don’t even want to imagine. It is not about “applications” like a faster algorithm or modeling the movement of a cell in water. It is about how many different differential structures there are of a 4D sphere. It is about finding the spaces where to find solutions of a system of multivariate conservation laws. It is about structure, it is about solving puzzles. Puzzles no one solved before. Puzzles no one knows how to solve. Puzzles for which you don’t even know if you have all the pieces.
It is impossible for mathematics to develop a sub-discipline which does not have any connection to math problems. It is even less likely to create a sub discipline which will stagnate. Because even if it is possible to close all the open problems of a sub-discipline, once there are no question or possibility of generalization (either less unlikely) people will move from there. Math is about the questions. Math is about the problems. Where there are not problems math do not dwell.
I think that I still don’t understand what you mean.
sounds like the culture of language or chess
You are correct.
I contest that Gödel was a philosopher. True, he made philosophy, but he was a mathematician at heart. Remember that he managed to found a solution of the differential equation describing general relativity where time is closed just like space…
This said, I agree that pure mathematics is mathematics for its own sake, but just in the sense that it tries to find answers for question that start in mathematics, as opposed to applied mathematics that tries to tind answers for question that start elsewhere.
Günter Ziegler said: “There’s applied Mathematics, and not yet applied Mathematics.”
Reblogged this on Trudge, Walk, Sprint, and Caper.
Thank you! Waking up this morning and reading an article from a guy married to woman mathematician and explaining why what she (and so I) does si so great!! It really brightens my day… It almost balances the fact that I have to finish writing a grant proposal. On the other hand, you reminded me of the latest Grey’s Anatomy episodes that I haven’t watched yet, not sure it’s going to help my grant proposal writing…
There is a fundamental misunderstanding here. Pure mathematics is necessary for applied mathematics to exist. The research of pure mathematics lays the foundations for applied mathematics, it’s just that some foundations haven’t been utilized yet.
Applied mathematics these days, from what I gather, mostly uses differential equations, which is like polynomials for calculus. Differential equations (or DE’s as I’ll refer to them from now on), being made from calculus, shares a parent with it. Namely, real analysis. Real and functional analysis abstracts the way we look at space, so that we can look at them as spaces per se, and not as spaces described by a particular differential equation. The set of solutions to a differential equation forms what’s called a topological vector space. It’s called a space because pure mathematics shows us that it’s -essentially- the same as one. We can treat it identically to 3D space, or, if it forms a space in some other dimension, look at it in the same way as some other dimension of space (1D, 2D, 5D, 12D, infinity D), only represented differently.
A topological vector space is just a certain type of vector space, which is a purely algebraic object. The point of vector space theory (called linear algebra) is to look at a vector space as an algebraic object per se, and not just one of the subspecies of vector spaces. Further, topological vector spaces have what’s called a topology, which generalizes the notion of distance, length, and orthogonality (what makes a right angle a right angle). So you can use topology to look at the distance and separability of objects per se, and leave all the other junk out of your thoughts.
All of this doesn’t even include how differential equations have Lie groups like polynomials have Galois groups, which I understand is totally meaningless to almost anyone without a math degree. Results from Galois theory, which is pure math, are used to motivate results in Lie theory, and find solutions of differential equations. But now I’m getting ahead of myself.
Every applied mathematician needs to know pure mathematics because they need to be able to look at their work from every direction. Sometimes it helps to look at the structure and ignore the shape, and vice versa. When an applied mathematican finds a new structure that they can apply to their work, it helps to have a ton of information about that structure so they don’t have to spend a lifetime figuring it out themselves. They can just look at the pure math literature and use the foundation that was built, maybe adding footnotes of their own along the way.
Disclaimer: I understand that some of my post will be offensive to the pedantic who are mathematically inclined. Please just take everything for the sake of argument and look at the big picture.
At least you said it! Too much information for the non-math public but it was needed.
What you are saying is by and large correct. However the thesis of some fairly heavy weight thinkers is that Pure Mathematics in places is no longer performing this task. Oswald Spengler, the German polymath, wrote a fair amount on this. He maintains, through the process of increasing specialization, it is possible for Maths sub-cultures to emerge that are so disconnected from reality they will never produce anything meaningful.
At the core of his argument is that Maths is invented not discovered, and for an invention to have meaning it must be connected to the greater culture in some way. He fully supports the idea of the most abstract of pure maths, provided the specialization producing it is still connected to real world; even if in the most distant and barely perceivable of senses.
A good summary of Spenglers “Math Factory” argument is given in Sal Restivo’s “The Social Life of Mathematics”, p10, Section V, http://logica.ugent.be/philosophica/fulltexts/42-2.pdf. As someone initially trained as a pure mathematician, then moving to applied maths, I find his arguments resonate with me.
Of course -some- subcultures in pure math can fail to produce anything meaningful, but so can -some- subcultures in applied math. The language of applied math is exactly as capable of producing useless mathematics. Pointing this out only in the case of pure math just adds to the pile of misleading argumentation in favor of applied math.
Bad applied mathematics is generally simply called bad applied mathematics. Irrelevant/bad ‘pure maths’ is treated differently and justified by the “it adds to our greater knowledge” principle.
Maybe I am starting to understand your point of view.
You are envious in my eyes of the attention pure math gets for producing what appears to be useless work.
If when we are talking about applied math we are talking about the same thing then in my mind it is obvious why applied math gets this treatment. In this world everything is about results, if your program is faster than before then it is good, and if someone made one even more faster then it is obsolete.
To produce noticeable results in pure math, or even better, to outdate some old results in pure math one has to produce a new theory with new insights that covers all the previous results as trivial. Moreover this theory has to be simple and easily teachable so that teaching the old one would be just a loss of time. As you can understand this is almost impossible.
When someone produce some interesting results in pure math he almost never will outdate old results, but most certainly will give new insights on the old subjects, and may be in other subjects. Even more, just the technique used in the proof of the new result may be valuable in other fields or branches.
You can understand that the way math is treated is different because of the difference it brings with itself.
One last thing: for no reason it will be disregarded if a branch of applied math gives new insights on math, or other branches. The problem is that it will give birth to a new field in pure math most probably.
As was already said: math answers well posed questions. To answer a question that is unanswered for a long time is difficult and the thinking needed to tackle the problem should not be trivial. As such it gets attention.
If you still don’t like the answer then I would like to hear an example of bad applied math and one of bad pure math.
I do not think you understand what I am saying.
Could you please give an example of what you are talking about? Some bad applied math and some bad pure math?
Interesting post, interesting to find out about pure and applied math. It’s good to know that whatever works have been done before, even though it was not applicable during a certain period, lead to something be created in the future.
Con Marlow e figli vi sentirete come si tratta solo di un altro negozio di alimentari di tutti i giorni ordinario ma attenzione perché l’apparenza inganna.
Thanks for sharing nice information.
“Q: You’re telling me there are people out there, right this instant, doing mathematics that may never, ever be useful to anyone?” – Is any mathematics useful to anyone? What is useful?
Real numbers are false, because they are not objects of nature, since they neither grow on trees nor can be mined from earth. Thus they are false and therefore they should not be used. If you do, then you will create a false thing, because false cannot be used to create truth. What is truth? Naturally, all objects of nature are truth, and all laws of nature are also truth.
Let us take one example. In engineering you will always find 3+4=5. Or something similar. All variables of nature or engineering have bounds, upper and lower. In the above example the bound is 5. No matter what the result is, it cannot go above 5. Just like all voltages and currents have a maximum allowable value. This makes math or algebra non-linear. But math assumes linearity. Thus no math can be used for engineering.
There is a similar problem with infinity. Many math theories, like Fourier Transform (FT) and Laplace Transform (LT) use infinity. No finite number can be an approximation for infinity. If you replace infinity by any finite number the above two theories will completely fail, their characteristics will dramatically change. As an example LT will not have any poles, FT will make uncertainty principle false. Thus all of math is false for nature, because they are all based on false thing, the real numbers. For more details take a look at https://theoryofsouls.wordpress.com/
Real numbers are false ?
No one claimed them to be true , I assume you are confusing the the numbers with dimensions and then comparing it with number system .Pure math is used to generalise the behaviour the numbers under very specific conditions and develop a framework or a system , by which we can predict the outcome accurately . Now this particular system can be applied to practical and realistic situations.
If you agree that real numbers are false, then how can you say you can create truth using false things like real numbers? Are you not contradicting yourself? I have given two examples in my first comment above. Do you think they are wrong? If yes, then explain why they are wrong? “Now this particular system can be applied to practical and realistic situations.” – Can you give an example where this math or any math can be used in practical and realistic situations?
I shall do this . First of all numbers can’t be true or false , this is very hard to explain but I will try . 1 is a number , 1 kg is the measure of weight . we use numbers to define and specify the characteristics of a particular object/thing/temperature/etc . Numbers cant be wrong or right , but the measure of the given object can . To be more clear , consider a statement ” All men have purple hair ” , while you can argue that this statement is right or wrong , but the word ” purple ” or ” hair ” can’t be proved to be true or false because it is a word , just a word which It doesn’t mean anything on its own and has no practical use . But you can combine words in a particular order or a specification with given set of ruleto get meaningful statements .
In Laplace and Fourier transformation part , your claims are not precise , so I have a vague idea but I don’t know what exactly you are trying to say , so I will talk about infinity instead . the statement ” no finite number can be a approximation for infinity ” is true , because approximation and finite contradict each other . we don’t aproximate finite with infinity , but the fact that you think we do is wrong . now this is because you dont know about “formal systems” . suppose ” x tends to infinity ” this does not mean that variable x reaches or tries to reach infinity nor is X exclusively a very large number , it simply means that there is no upper limit to the value of X and it can be any number from the given set and if the set is not defined we assume it to be set of real numbers .
system and framework : ~ it can be a function , a group , a inequality , a statement , a pattern , etc . Anything which follows certain rules and gives us a desired outcome.
Practical uses of pure math .
If pure math is practically used then it is no longer considered pure math , it is called applied math . For example cryptology . digital encryption uses Diophantine equations and various algorithms to generate very specific numbers which satisfy a given criteria , most likely it will be a prime and it will be very large. to be precise they used to scramble your data into a gibberish form and then they transmit it , only the intended receiver can rebuild the data to its origanal form . Cryptology uses a lot of number theory . Now these algorithms and eqns were completely irrelevant and pure less than two decades ago .
Conclusion : ~
There is no distinct difference between pure and applied math . Math which can be practically used is called applied math .The most popular misconception is that ” pure math is useless , because we can’t use it . ” , we can’t use it because we don’t know how to , but the fact that it is has a practical application is ignored.
Please go learn about number system and formal systems , before making such statements about , real numbers being false and proving it by using a function which transforms integrals into a complex functions to find the anti derivatives.
“First of all numbers can’t be true or false.” – I have defined what is true and false. You have probably missed the definition on my first comment. If something is not an object of nature then it is false. In our society we have created two objects, real numbers and money. Both are false. Therefore any applications of money and real numbers will be false. Therefore, math, physics, and economics will be false. You cannot create truth using false things.
Laplace Transform (LT) is a definite integral. I am not sure if you can consider it as an anti-derivative. It is a process where you eliminate one of the variables of a kernel. But what you produce is wrong, because infinity does not exist in nature. If you replace infinity by any finite number in LT integral, the LT will change completely; LT will become an analytic function. Thus LT will create a false application, a complex function with poles.
Since our society is created by money it is a false society. Cryptography is thus a false application. Just like tax and debts are false things in our society. Remove money, all these false subjects will vanish. Take a look at money-less economy (MLE) chapter in the free book on Soul Theory at https://theoryofsouls.wordpress.com/category/c-ch3-moneyless-economy/ , if you think money is not false. Note that money is a real number also.
Are natural numbers truth?
“Are natural numbers truth?” – No, natural numbers are not truth, because they are not objects of nature. To be able to do some math with natural numbers, they must satisfy some properties, which are also not valid as laws of nature. Natural numbers have an order on real line. There is no such order in nature. You cannot say apple is greater than orange. No two objects in nature are identical. All objects of nature are continuously changing; they are alive. All objects of nature are simultaneously and continuously interactive with each other. Our mathematics cannot comprehend such properties of objects of nature. Math is too primitive for any applications of nature and engineering.
Do we need to? Do we need to differentiate between an apple and an orange when giving people fruit? Do we need to have some distinction between two perfectly eatable apples?
The word engineering exists and is possible only because mathematics aids our rational thinking. It seems preposterous to say that Math is too primitive for ANY application of engineering.
There is no order in nature? So I am not writing this post after you? Is the concept of “after” false? My parents did not live before me? Is it devoid of meaning the sentence “the sun is bigger that the earth”?
Are comparative adjective false then?
It seems to me that you use false and true arbitrarily. We are objects of nature. We are true. We made houses. Houses are true. We made money. Money is false.
Somewhere in between our actions and our way of doing things contradicts naturality and loses truth value? Everything we do is made by nature using us. So it should be true.
If not, when exactly thing start becoming false? When a monkey starts using a rock to open a coconut, does in that moment the rock become false?
I still can’t follow your reasoning.
“The word engineering exists and is possible only because mathematics aids our rational thinking.” – That is not a correct concept. None of the following famous people, pioneers of our modern society, can be considered formally educated – Bill Gates (software), Steve Jobs (software and hardware), Wright Brothers (Airplane), Benjamin Franklin (business man, scientist, politician, revolutionary), Graham Bell (electrical communication), Thomas Edison (electrical power) etc. So education is not necessary to produce engineering products.
On the contrary, engineering has become, unstable, unreliable, because of applications of false math and physics. Our engineering is polluting our environment because of poor science and economic theories, both of which use real numbers and money. Money is also a real number.
We did not make real numbers and money. They are not physical objects. Arthur George was a king. Here king is a label for George, king is not an object, George is the object. Same is true for the statement – Apple is $10. Money value is a label.
“Our engineering is polluting our environment.”
saying that via a computer is funny.
Ok, you are what is called mathematical fictionalist.
Never thought you really existed. The need to require a meaning for the sentence “this apple is true.” is beyond me, but it is a free world till the moment you manage public money and close universities because of your beliefs.
I did not say that – close universities. Remove money from the economy, all problems will be solved. Poverty, unemployment, wars, religions, pollution, ignorance – all will vanish. Heaven will come to earth. Take a look at the money-less economy (MLE) chapter at https://theoryofsouls.wordpress.com/category/c-ch3-moneyless-economy/
> real numbers are false
> money is false and a problem
> in short , anything which is not an object nature is false
> your name is not an object of nature , thus it is false
> a marriage between two humans , is not a object of nature thus it is false .
> love , or emotions are not an object of nature thus , they are false .
Do you seriously want me continue , or are you(indnsp) satisfied ?
I think I have also mentioned that objects of nature obey the laws of nature. All objects of nature have many characteristics, which may be considered as laws of nature also. For example Oxygen is an object of nature. It has a specific characteristic like 8 proton, 8 electrons, etc. Oxygen can also be described by the ways it interacts with other elements of nature to create new compounds. All these characteristics can be also considered as laws of nature. Thus truths are only objects of nature and the laws of nature.
Now it is clear that love, emotions are characteristics of objects of nature, like humans, animals, plants etc. Similarly marriage or sexual relations are laws of nature. Thus these are all truths. Now is name a truth? I think it is a law of nature too. There are about one million palm leaf booklets in India, which describe the destiny of that many people living now in the world. These books were written at least 10 thousand (maybe even 100,000) years before. These books give the names of each person. There is a family in USA, called Leininger, who named his son as James. When James learned to speak he talked about his past life, one surprising thing was that his name in the previous life was also James. For more details, you can take a look at the destiny and reincarnation chapters in the free book at https://theoryofsouls.wordpress.com/
What the hell are you talking about? Almost all physical Sciences build from pure math. Pure math helps applied math/physics and that in turn creates new technologies and engineering. Without pure mathematics, there would be no calculus and algebra, which in turn would mean no Nasa, no computers, no tall buildings, no electronics, no cars, no planes, no Einstein’s theories of relativity (Pure mathematics Geometry), no imaging machines, no Photography. EVERYTHING builds from pure Mathematics research.
Hmm…
Calculus and algebra date to long before there was any clear distinction between “pure” and “applied.” Newton, for example, developed Calculus to solve very specific applied problems. It’s purification (through 19th century analysis) came much later.
Einstein’s theory of relativity is a stronger example of pure math enriching the soil for applications to grow.
As for “almost all” physical sciences being grounded in pure math, I don’t really see it!
For Ben Orlinon October 30, 2015 at 4:40 pm
“Newton, for example, developed Calculus to solve very specific applied problems.” Did Newton really produce anything meaningful?
All theories have the following structure: (1) A set of assumptions (2) A set of results and (3) A statement that says items in two will be valid only when items in one are valid.
Clearly nature does not and cannot make any assumptions. Therefore all theories of science must be wrong. Thus entire physics and mathematics cannot be used in engineering and for the description of nature. Let us take Newton’s first law – An object will continue in motion in a straight line with constant velocity. Have you ever seen such an object on earth or in space? No, we didn’t. You can then see we are teaching false physics to our students.
Why did Newton fail here? Because he assumed – isolated environment – which is impossible in nature.
For Sulinder on October 30, 2015 at 4:24 pm
How do you know that math “creates new technologies and engineering”? Who told you that?
As I have already mentioned none of the following famous people of USA, pioneers of our modern society, can be considered formally educated – Bill Gates (software), Steve Jobs (software and hardware), Wright Brothers (Airplane), Benjamin Franklin (business man, scientist, politician, revolutionary), Graham Bell (electrical communication), Thomas Edison (electrical power) etc. So math and not even any education is necessary to produce engineering products.
“EVERYTHING builds from pure Mathematics research”. – is an absolutely wrong concept. Take a look at many math and physics examples here https://theoryofsouls.wordpress.com/ to see why they are all wrong.
Pure Math provides a true (i.e. logically consistent) theoretical framework that gives confidence to applied math techniques. Pure Math also often provides practical applications; e.g. Number Theory regarding Prime Number Behavior yielded our modern Public/Private Key encryption techniques that underpins transactions on the Internet.
Or, think of it this way.
You wanna do something. I don’t know. Get a job maybe. Or become famous or get something to eat. You’re not sure what. But if you don’t get up off the couch, turn off “Ellen” and get out of the house and start the adventure, you’ll never get there, wherever or whatever “there” is. Nothing moves forward unless we move it forward. We don’t know what’s next, but if we keep looking, we might just find it. Or not, but we’ll find something.
A bit late. . . . Pure mathematics, or perhaps better called fundamental mathematics, is just that pure (with no immediate application in mind, rather just because it is there – kind of like Mt Everest) and at times fundamental to applied mathematics as has been so aptly said above. One can construct an algorithm to solve a real-world problem (applied mathematics), but it would sure be nice to be able to prove (pure mathematics) to the world that it converges in a reasonable amount of time. This is so we all just do not sit around twiddling our thumbs hoping for Moore’s Law to kick in many, many times only to find out the algorithm is NP-Complete and it “ain’t worth waitin’ for” in the version proposed, We need more theory to design a better algorithm and more theory to prove it converges in a reasonable amount of time.
You do not have an understanding of mathematics. At the heart of most pure mathematics is computation. Pure mathematics forms the structure for applied mathematics. For example, an applied mathematician will still use manifolds, care about Complexity theory and want a solution to a PDE.
It is sad that pure and applied mathematics have been separated (just like how physics has been separated from both), but this mostly stems from applied mathematicians not being able to understand pure mathematics and pure mathematicians from finding applied mathematics not worth their time since most of it is grunt work.
The frame work of pure mathematics, such set theory or model theory, is not meant to decide if everything is true or false, but to model mathematics. Set theory was made to deal with problems of infinity, and it just so happened that things such as the continuum hypothesis did not match will this structure, but as far as man can tell not, such a statement does not have bearing on active mathematics.